SSC CGL 2017: Quantitative Aptitude Quiz – Geometry & Trigonometry

June 8, 2017

Hello and welcome to exampundit. Here is the very first set of SSC CGL Quantitative Aptitude Quiz for 2017 SSC CGL.

(adsbygoogle = window.adsbygoogle || []).push({});

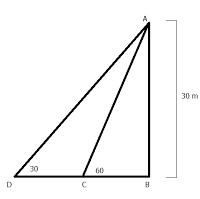
1. Saurabh was standing in his society’s apartment at point D and watching the top of his building, which makes an angle of elevation of 30 º. Saurabh then walked some distance towards his building and then his angle of elevation to the top of tower becomes 60 º. If the height of the building is 30 meters, find the distance he moves.
a) 22m
b) 22√3 m
c) 20m
d) 20√3 m

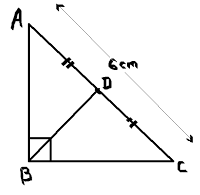
2.In ∆ ABC, <B is a right angle. AC is 6 cm. D is the midpoint of AC. Find the length of BD.

a) 4 cm
b) √6 cm
c) 3 cm
d) 3.5 cm

3.Three circles touch each other externally. The distance between their centers is 5 cm, 6 cm, and 7 cm. Find the radii?
a) 2,3,4
b) 3,4,1
c) 1,2,4
d) None

4.If 3 tan θ+ 4 = 0 , and Π /2< θ <Π , then find 2 cotθ -5 cosθ + sinθ ?
a) -53/10
b) 7/10
c) 23/10
d) 37/10

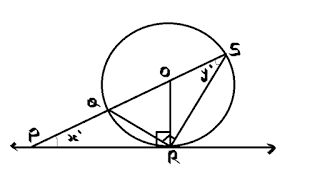
5. PTis a tangent of a circle with center O at point R. If the diameter SQ is extended it meets PT at point P. If <SPR=x º and <QSR=Y º, what will be the value of x º +2y º?

a) 90
b) 135
c) 105
d) 180

6.If tan ( x+y) . tan (x-y)=1 , find the value of tan (2x/3) .
a) 1/√ 3
b) 2/√ 3
c) √3
d) 1

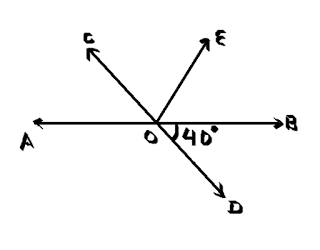
7.AB and CD intersect at O. <AOC+<BOE=70 º and <BOD =40 º . Find the reflexive <COE.

a) 110 º
b) 80 º
c) 70 º
d) 250 º

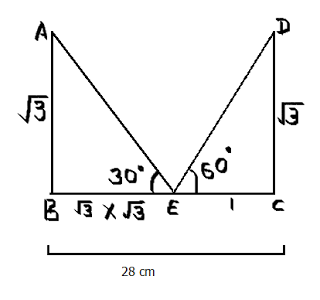
8.Two towers of equal height are standing opposite to each other on either side of a pavement which is 28 meters wide. From a point between them on the road, the angles of elevation of tops of the towers are 30 º and 60 º respectively. Find the height of the tower.
a) 6√3
b) 5√3
c) 4√3
d) 7√3

9.The value of 3cos80 º cosec 10 º + 2 cos 59 º cosec 31 º is :
a) 1
b) 3
c) 2
d) 5

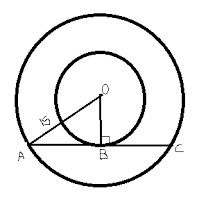
10.The radii of two concentric circles are 9 and 15 cm. The chord of a larger circle is a tangent to the smaller circle then the length of the chord is:
a) 24
b) 12
c) 30
d) 18

Answers & Explanations

1.D

Tanθ = p/b
Tan 30 º =1 / √3 tan 60 º = √3
Tan 30 º =AB/DB Tan 60 º =AB/BC
DC=DB-BC
=AB / tan 30 º – AB /tan 60 º
= 30√ 3 – 30 / √3 = (90-30)/ √3 =60 / √3 = (3×20)/ √3 = 20√ 3

2. C
In Right angled ∆, the length of median to the hypotenuse is half of the hypotenuse = 6/2 = 3 cm

3. A

Let the radii of the circles be x, y, and Z
Then we have x+y=5
X+z=7
Y+z=6
Adding all we get
2( x+y+z)=5+7+6=18
=>x+y+z=9
5+z=9=>z=4
7+y=9=>y=2
X+z=7=>x=3
Answer is 3,4,2

4. C

3 tanθ +4=0=>tanθ = -4/3
Hence perpendicular=4, base = 3 and hence hypotenuse = 5( by Pythagoras theorem or trigonometric triplets)
Π /2< θ <Π =2nd quadrant
Hence sin is +ve and cot and cos are negative
2cotθ -5cosθ+sinθ=2(-3/4)-5(-3/5)+4/5=23/10

5. A

In ∆ORS, OR=OS=radii
Hence in isosceles triangle <ORS=<OSR=y º
<POR=y+y=2y ( external angle)
In POR, 2y º +x º + 90 º = 180 º
=>2y º +x º =180 º -90 º =90 º

6. A
Tan (x+y)=1/tan(x-y)=cot(x-y) = tan [90-(x-y)] => x+y=90-x+y
=>2x=90
=>2x/3=30
Tan 30 = 1/ √3

7. D

<AOC=<BOD=40 º
<BOE=40 º
Reflexive <COE=<COD+<DOB+<BOE=180 º+40 º+30 º=250 º

8. D